When we say that something is conserved, we mean that it remains constant. If we have a certain amount of a conserved quantity at some instant of time, we will have the same amount of that quantity at a later time. This does not mean that the quantity cannot change form during that time, but if we consider all the forms that the quantity can take, we will find that we always have the same amount.

 

An example of a conserved quantity that you are already familiar with is mass. For instance, imagine that a light bulb is dropped on the floor and shatters into many pieces. No matter how the bulb shatters, the total mass of all of the pieces together is the same as the mass of the intact light bulb, because mass is conserved.

The concept of work and the work-energy theorem have led us to the conclusion that an object can possess two kinds of energy: kinetic energy, KE, and gravitational potential energy, PE. The sum of these two energies is called the total mechanical energy E, so that E = KE + PE. Later on we will update this definition to include other types of potential energy in addition to the gravitational form. The concept of total mechanical energy will be extremely useful in describing the motion of objects.

By rearranging the terms, the work-energy theorem can be expressed in terms of the total mechanical energy:

$$W_{NC}= \Delta KE + \Delta PE$$ $$W_{NC}= (KE_f - KE_i) + (PE_f - PE_i)$$ $$W_{NC}= (KE_f + PE_f) - (KE_i + PE_i)$$ $$W_{NC}= E_f - E_i$$

This is just another form of the work-energy theorem. It states that $W_{NC}$, the net work done by external nonconservative forces, changes the total mechanical energy from an initial value of $E_0$ to a final value of $E_f$.

If we can ignore friction and other nonconservative forces, or if only conservative forces do work on a system, we arrive at a particularly simple and beautiful relation involving energy:

$$E_f = E_i = constant$$

This is the principle of conservation of mechanical energy for conservative forces:

If only conservative forces do work, the total mechanical energy of a system (KE + PE) neither increases nor decreases in any process. It stays constant—it is conserved.

We have seen that kinetic energy can be converted into gravitational potential energy and vice versa. In general, energy of all types can be converted from one form to another.

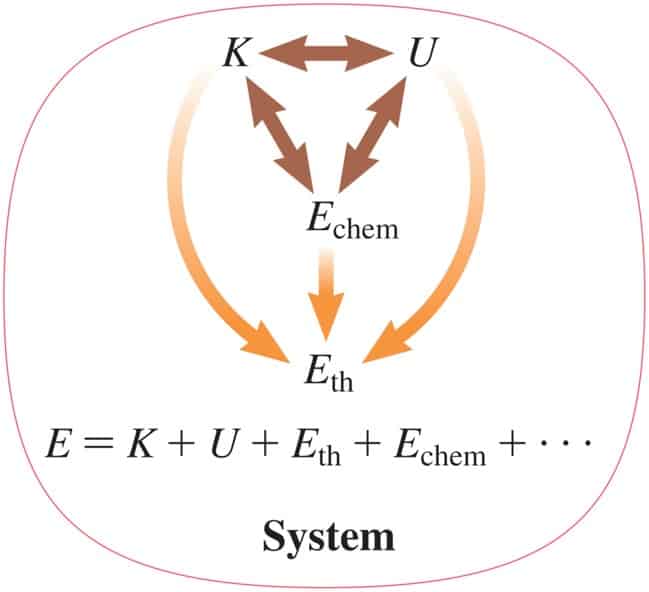
One of the great results of physics is that whenever energy is transferred or transformed, it is found that no energy is gained or lost in the process.

This is the law of conservation of energy, one of the most important principles in physics; it can be stated as:

The total energy is neither increased nor decreased in any process. Energy can be transformed from one form to another, and transferred from one object to another, but the total amount remains constant.

We have already discussed the conservation of energy for mechanical systems involving conservative forces, and we saw how it could be derived from Newton’s laws and thus is equivalent to them. But in its full generality, the validity of the law of conservation of energy, encompassing all forms of energy including those associated with nonconservative forces like friction, rests on experimental observation. Even though Newton’s laws are found to fail in the submicroscopic world of the atom, the law of conservation of energy has been found to hold in every experimental situation so far tested.

As discussed in the previous articles, the total linear momentum is conserved when two objects collide, provided they constitute an isolated system. When the objects are atoms or subatomic particles, the total kinetic energy of the system is often conserved also. In other words, the total kinetic energy of the particles before the collision equals the total kinetic energy of the particles after the collision, so that kinetic energy gained by one particle is lost by another.

Collisions are often classified according to whether the total kinetic energy changes during the collision:

1. Elastic collision: One in which the total kinetic energy of the system after the collision is equal to the total kinetic energy before the collision.

2. Inelastic collision: One in which the total kinetic energy of the system is not the same before and after the collision; if the objects stick together after colliding, the collision is said to be completely inelastic.

Collisions in Two Dimension

The collisions discussed so far have been head-on, or one-dimensional, because the velocities of the objects all point along a single line before and after contact. Collisions often occur, however, in two or three dimensions. For example, a two-dimensional case in which two balls collide on a horizontal frictionless table.

Work and energy are among the most fundamental and useful concepts in physics. So far we discusses their application to translational motion. These concepts are equally useful for rotational motion, provided they are expressed in terms of angular variables.

Rotational Work done by a constant torque

$$ W_{Rotational} = \tau \theta$$

Rotational Kinetic Energy and The Work-Energy Theorem

$$ KE_{Rotational} = \dfrac{1}{2} I \omega^2 $$

Total Mechanical Energy of a rotating body (combined translational and rotational motion)

$$E = \dfrac{1}{2} m v^2 + \dfrac{1}{2} I \omega^2 + mgh$$