A 1.0-cm-radius hose with a 0.50-cm-radius exit nozzle is being used to fill a 1,000-ml beaker with oil (1,000 ml = 0.0010 m3). The velocity of the oil in the hose is v = 0.40 m/s as shown in the figure. The density of the oil is 960 kg/m3, and the atmospheric pressure is 1.01 × 105 Pa.

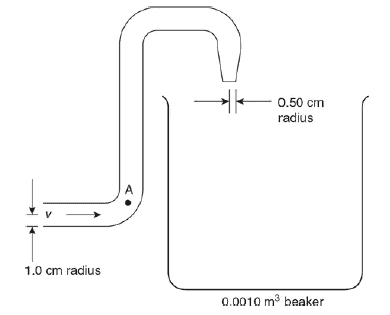
(a) The nozzle attached to the end of the hose has a smaller radius than the hose. If the nozzle is removed from the hose, will the beaker be filled faster? Justify your answer with conservation laws.

(b) Calculate the exit velocity of the oil from the nozzle.

(c) How long will it take to fill the beaker?

(d) Point A is shown in the figure. How does the pressure in the fluid at point A compare to the pressure in the fluid at the exit nozzle? Justify your claim.

(e) The hose is now used to fill a 200-ml graduated cylinder with oil to the same height as the height of the oil in the 1,000-ml beaker. Compare the net force from the oil on the bottom of the 200-ml cylinder and the 1,000-ml beaker. Explain your answer.

(f) A cube of lead with a side dimension of 5.0 cm is slowly lowered into the beaker of oil by a thin string attached to a spring scale at a constant rate, as shown in the figure. The density of lead is 11,300 kg/m3.

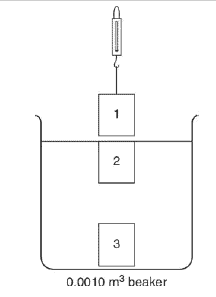
i. What will be the spring scale reading in newtons when the lead has been submerged to location 2?

ii. Does the spring scale reading increase, decrease, or stay the same when the cube is lowered from location 2 to location 3? Justify your answer by referencing the pressure of the fluid on the lead cube.

iii. The lead cube is lowered from above the oil’s surface (location 1) to a spot just below the surface (location 2) until the cube is just above the bottom of the beaker (location 3). Describe any changes in pressure on the bottom of the beaker during this process. Explain your answer.