The Refraction of Light: Lenses and Optical Instruments
This change in direction is called refraction. To describe the extent to which the speed of light in a material medium differs from that in a vacuum, we use a parameter called the index of refraction (or refractive index). The index of refraction is an important parameter because it appears in Snell's law of refraction, this law is the basis of all the phenomena discussed in this article.
Digital lesson: What Are Some Properties Of Light?
|
Digital lesson: Mirrors And Lenses
|
|---|
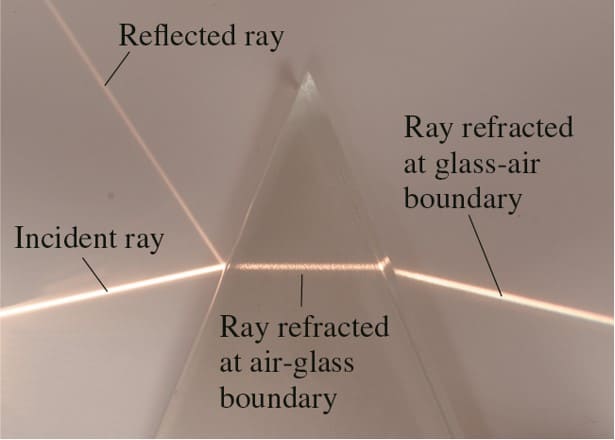
Snell's Law and the Refraction of Light
When light strikes the interface between two transparent materials, such as air and water, the light generally divides into two parts. Part of the light is reflected, with the angle of reflection equaling the angle of incidence. The remainder is transmitted across the interface. If the incident ray does not strike the interface at normal incidence, the transmitted ray has a different direction than the incident ray. When a ray enters the second material and changes direction, it is said to be refracted.
Total Internal Reflection
When light passes from a medium of larger refractive index into one of smaller refractive index for example, from water to air, the refracted ray bends away from the normal. As the angle of incidence increases, the angle of refraction also increases. When the angle of incidence reaches a certain value, called the critical angle $\theta_c$, the angle of refraction is 90°. Then the refracted ray points along the surface. When the angle of incidence exceeds the critical angle, there is no refracted light. All the incident light is reflected back into the medium from which it came, a phenomenon called total internal reflection. Total internal reflection occurs only when light travels from a higher-index medium toward a lower-index medium. It does not occur when light propagates in the reverse direction-for example, from air to water.
Apparent Depth
One interesting consequence of refraction is that an object lying under water appears to be closer to the surface than it actually is.
The Displacement of Light by a Transparent Slab of Material
A windowpane is an example of a transparent slab of material. It consists of a plate of glass with parallel surfaces. When a ray of light passes through the glass, the emergent ray is parallel to the incident ray but displaced from it.
Polarization and the Reflection and Refraction of Light
For incident angles other than 0°, unpolarized light becomes partially polarized in reflecting from a nonmetallic surface, such as water. To demonstrate this fact, rotate a pair of Polaroid sunglasses in the sunlight reflected from a lake. You will see that the light intensity transmitted through the glasses is a minimum when the glasses are oriented as they are normally worn. Since the transmission axis of the glasses is aligned vertically, it follows that the light reflected from the lake is partially polarized in the horizontal direction.
The Dispersion of Light: Prisms and Rainbows
When the light enters the prism at the left face, the refracted ray is bent toward the normal, because the refractive index of glass is greater than that of air. When the light leaves the prism at the right face, it is refracted away from the normal. Thus, the net effect of the prism is to change the direction of the ray, causing it to bend downward upon entering the prism, and downward again upon leaving. Because the refractive index of the glass depends on wavelength, rays corresponding to different colors are bent by different amounts by the prism and depart traveling in different directions. The greater the index of refraction for a given color, the greater the bending. If a beam of sunlight, which contains all colors, is sent through the prism, the sunlight is separated into a spectrum of colors. The spreading of light into its color components is called dispersion.
Animated Physics: Refraction and Total Internal Reflection
|
Interactive Demonstration: Snell's Law
|
Interactive Demonstration: Critical Angle
|
|---|---|---|
Virtual Investigations: Snell’s Law
|
Digital simulations: Refraction and Total Internal Reflection
|
Digital simulations: The Dispersion of Light
|
Digital lesson: Mixing Colors
|
Digital Figure: car’s interior rearview mirror
|
Lenses
The lenses used in optical instruments, such as eyeglasses, cameras, and telescopes, are made from transparent materials that refract light. They refract the light in such a way that an image of the source of the light is formed.
The Formation of Images by a Converging Lens
Three specific paraxial rays are especially convenient to use in the ray-tracing method, and these three rays leave from a point on the top of the object.
The Formation of Images by a Diverging Lens
The same three rays are used.
Digital lab: Images From Convex Lenses
|
Animated Physics: Lenses
|
Concept Map: Images
|
|---|---|---|
Virtual Investigations: Ray Tracing for Lenses
|
Digital simulations: Mirrors and Lenses
|
Digital Interactives: Optics
|
The Thin-Lens Equation and the Magnification Equation
For an object placed in front of a lens, Snell's law of refraction leads to the technique of ray tracing and to equations that are identical to the mirror and magnification equations. Thus, mirrors work because of the reflection of light, whereas lenses work because of the refraction of light, a distinction between the two devices that is important to keep in mind.
Interactive Demonstration: Lenses
|
|---|
Lenses in Combination
Many optical instruments, such as microscopes and telescopes, use a number of lenses together to produce an image. Among other things, a multiple-lens system can produce an image that is magnified more than is possible with a single lens.
The Human Eye
Without doubt, the human eye is the most remarkable of all optical devices.
The Refractive Power of a Lens-The Diopter
The extent to which rays of light are refracted by a lens depends on its focal length. However, optometrists who prescribe correctional lenses and opticians who make the lenses do not specify the focal length directly in prescriptions. Instead, they use the concept of refractive power to describe the extent to which a lens refracts light.
Angular Magnification and the Magnifying Glass
If you hold a penny at arm's length, the penny looks larger than the moon. The reason is that the penny, being so close, forms a larger image on the retina of the eye than does the more distant moon. The brain interprets the larger image of the penny as arising from a larger object. The size of the image on the retina determines how large an object appears to be. However, the size of the image on the retina is difficult to measure. Alternatively, the angle $\theta$ subtended by the image can be used as an indication of the image size. The angle $\theta$ is called the angular size of both the image and the object. The larger the angular size, the larger the image on the retina, and the larger the object appears to be.
The Compound Microscope
To increase the angular magnification beyond that possible with a magnifying glass, an additional converging lens can be included to "premagnify" the object before the magnifying glass comes into play. The result is an optical instrument known as the compound microscope. (
The Telescope
A telescope is an instrument for magnifying distant objects, such as stars and planets. Like a microscope, a telescope consists of an objective and an eyepiece (also called the ocular). When the objective is a lens, the telescope is referred to as a refracting telescope, since lenses utilize the refraction of light.*
Lens Aberrations
Rather than forming a sharp image, a single lens typically forms an image that is slightly out of focus. This lack of sharpness arises because the rays originating from a single point on the object are not focused to a single point on the image. As a result, each point on the image becomes a small blur. The lack of point-to-point correspondence between object and image is called an aberration.
Digital lesson: Light Waves And Sight
|
Digital Figure: The Human Eye
|
|---|
You don`t have permission to comment here!
Report